Standing and Progressive Waves - Learn

Standing and Progressive Waves

Progressive Waves:

A mechanical wave in a medium is caused by an input of energy (soundwave and waterwave). This will cause the particles of the medium to oscillate. The disturbance subsequently travels through a medium transporting energy as it moves. For example, in a water wave, as the wave travels, a series of crests and troughs appear to travel across the surface. This type of wave pattern is sometimes referred to as a travelling wave.

Progressive waves are observed when a wave can move along or through a medium, that is, it is not confined to one position. The most common progressive waves we can observe are ocean waves.

Standing Waves:

A standing wave is a wave that displays a wavelength and an amplitude but it does not appear to be moving. These waves are often referred to as stationary waves.

If a rope is fixed at one end to a wall and vibrated once, you will observe a wave travel along the the rope, reflect off the fixed position and travel back. If the rope was vibrated twice, the first wave will reflect off the fixed position and interact with the second wave. These two vibrations or waves will interfere with each other and superimpose, creating a resultant wave.

If the rope in the example above is vibrated at just the right frequency, the interference of the incident wave and the reflected wave occur in such a manner that there are specific points along the medium that appear to be standing still. We refer to these points as nodal points (nodes) and the pattern is often called a standing wave pattern. The displacement of all other points along the medium change with time, but in a regular manner. These points vibrate back and forth from a positive displacement to a negative displacement with their own specific amplitude. Positions where a maximum displacement occurs are referred to as antinodes. Even though the standing wave appears to not be moving, it is important to remember that the standing wave is the superposition of two travelling waves moving in opposite directions. This pattern is illustrated below:

The purple line represents the ropes displacement at an instant in time and the orange line represents the displacement half a period later ( $\cfrac { T }{ 2 }$ ). The distance between consecutive nodes or antinodes is half a wavelength ( $\cfrac { \lambda }{ 2 }$ ).

Wave equations and relationships can all be used to analyse standing waves:

$v=f\lambda$ ( $f$ is the frequency required to achieve the standing wave pattern).

$f=\cfrac { 1 }{ T }$

$T=\cfrac { 1 }{ f }$

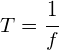
Example 1:

Outline an example of where a standing wave could be observed:

Answer:

Stringed instruments (guitars, violins) form standing waves. The strings are fixed at both ends and when a string is played the strings vibrate in such a way that standing waves form between these fixed points.

Wind instruments (trumpets) form standing waves in the air columns of the instrument.