Projectile Motion Problems - Learn - ScienceFlip

Projectile Motion Problems – Learn

There is a large number of possible Projectile motion problems you may be required to solve. They can vary in the type of information you are given, the questions that you are asked and also the flight of the projectile. The projectile problem may involve:

Projectiles that launch horizontally or vertically
Projectiles that launch and land at the same height
Projectiles that launch and land at different heights
Projectiles that hit a boundary (cliff or building)

Projectile motion problems will usually require you to answer multiple parts or solve a problem using several steps. In any case, developing a method or technique you can use for every problem allows you to become more confident in completing problems.

General technique for analysing projectile motion problems:

Read through the question and draw a diagram that reflects the motion of the projectile (some questions may give you a diagram)
Fill in any information on your diagram that you can determine from the question (initial velocity, launch angle, height of cliff, etc)
Denote a direction as positive
Write a list of the variables you have been given in the question
For each part of the question, write the variable you are trying to determine
Determine the equation you will use to solve the problem

Example 1:

A projectile is hit with an initial velocity of 75m/s at angle of 30º to the horizontal. Determine:

a) How long will the projectile stay in the air (assume it strikes the ground at the same height it was launched)

b) How far will the projectile go before hitting the ground?

c) What will be the maximum height of the projectile?

Answers:

Note: down is positive

$a=9.8\:{ m }/{ { s }^{ 2 } }$
$u=75\:{ m }/{ s }$
${ u }_{ x }=75\cos { 30 } =64.95\:{ m }/{ s }$
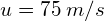
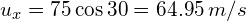
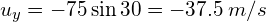${ u }_{ y }=-75\sin { 30 } =-37.5\:{ m }/{ s }$ (down is positive)

a) Time of flight = 2× time to reach maximum height

using: ${ v }_{ y }={ u }_{ y }+{ a }_{ y }t$

where:

${ v }_{ y }=0$ (top of flight)
${ u }_{ y }=-37.5\:{ m }/{ s }$
$a=9.8\:{ m }/{ { s }^{ 2 } }$

$0=-37.5+9.8t$

$37.5=9.8t$

$t=\cfrac { 37.5 }{ 9.8 }$

$t=3.8265\:sec$

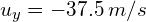
Therefore, time of flight = $2\times 3.8265=7.65\:sec$

b) using: $s={ u }_{ y }t+\cfrac { 1 }{ 2 } a{ t }^{ 2 }$

where:

${ u }_{ x }=64.95\:{ m }/{ s }$
$a=0\:{ m }/{ { s }^{ 2 } }$
$t=7.65\:sec$

$\Delta x=64.95\times 7.65+\cfrac { 1 }{ 2 } (0)(7.65)^{ 2 }$

$\Delta x=496.87\:m$

c) using: ${ v }_{ y }^{ 2 }={ u }_{ y }^{ 2 }+2as$

where:

${ v }_{ y }=0\:{ m }/{ s }$
${ u }_{ y }=-75\sin { 30 } =-37.5\:{ m }/{ s }$
$a=9.8\:{ m }/{ { s }^{ 2 } }$

${ 0 }^{ 2 }={ -37.5 }^{ 2 }+2\times 9.8\times \Delta y$

${ 0 }=1406.25+19.6\Delta y$

$\cfrac { -1406.25 }{ 19.6 } =\Delta y$

$\Delta y=-71.75\:m$

As down is positive, the maximum height = $71.75\:m$

Example 2:

A rock is thrown at a cliff 20m away with an initial velocity of 40m/s at angle of 25º to the horizontal. Determine:

a) How long will it took for the rock to hit the cliff:

b) At what height did the rock strike the cliff?

Answers:

Note: down is positive

$a=9.8\:{ m }/{ { s }^{ 2 } }$
$u=40\:{ m }/{ s }$
${ u }_{ x }=40\cos { 25 } =36.25\:{ m }/{ s }$
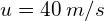
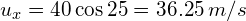
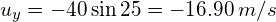${ u }_{ y }=-40\sin { 25 } =-16.90\:{ m }/{ s }$ (down is positive)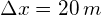
$\Delta x=20\:m$

a) using: $s={ u }_{ x }t+\cfrac { 1 }{ 2 } { a }_{ x }{ t }^{ 2 }$

where:

${ u }_{ x }=36.25\:{ m }/{ s }$
$a=0\:{ m }/{ { s }^{ 2 } }$
$t=7.65\:sec$
$\Delta x=20\:m$

$20=36.25t+\cfrac { 1 }{ 2 } \times 0\times { t }^{ 2 }$

$t=\cfrac { 20 }{ 36.25 }$

$t=0.55\:sec$

b) using: $s={ u }_{ y }t+\cfrac { 1 }{ 2 } a{ t }^{ 2 }$

where:

${ u }_{ y }=-16.90\:{ m }/{ s }$
$t=0.55\:sec$
$a=9.8\:{ m }/{ { s }^{ 2 } }$

$\Delta y=-16.90\times 0.55+\cfrac { 1 }{ 2 } ({ 9.8 })(0.55^{ 2 })$

$\Delta y=-7.81\:m$

As down is positive, the height the rock strikes the cliff= $7.81\:m$