Forces and Circular Motion – Learn
In this section we will consider the forces acting on an object executing uniform circular motion by analysing the following three situations:
- car moving around horizontal circular bends
- a mass on a string
- objects on banked tracks
Car moving around horizontal circular bends
As a vehicle makes a circular turn, the inertia of the vehicle means that it wants to move along a straight line at a tangent to the curve. However, the vehicle will remain in uniform circular motion due to the friction between the tyres and the road. This frictional force is the centripetal force that allows the car to turn the corner: ![]()
Questions may vary in the way the frictional force is presented. It may be a constant force or determined from the coefficient of friction, ![]()
- If the frictional force,
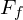 , is a constant, then:
, is a constant, then: 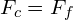
- If the frictional force is determined from the coefficient of friction, then:
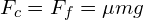
A mass on a string
Uniform circular motion can also occur when a mass on the end of a string is swung around in a circle at an angle from a fixed point. The string will have a tension force that allows this to occur. The object itself is undergoing uniform circular motion and so the net force on the object is equal to the centripetal force: ![]() . The centripetal force is the resultant force of the weight force and the tension in the string:
. The centripetal force is the resultant force of the weight force and the tension in the string: ![]() .
.
The triangle that forms as a result of ![]() ,
, ![]() and
and ![]() can be used to analyse lengths/distances and forces by applying trigonometry.
can be used to analyse lengths/distances and forces by applying trigonometry.
Objects on banked tracks
Banking a track or a road is achieved by tilting the surface up toward the centre of the circle. Velodromes and some roads are designed this way to allow for greater speeds and safety when turning. Banking allows some of the reaction force to contribute to the net force acting on a vehicle. This increases the magnitude of the net force and therefore, the magnitude of the centripetal force acting on the vehicle allowing increased speeds to be safely achieved.
*note: friction plays a big role in any vehicle turning a corner and many problems of this nature will not consider the role of friction. Problems become more complex when friction is considered but can be broken down by analysing the vertical and horizontal components of friction.
Banked tracks (ignoring friction):
The diagram below represents the forces acting on a vehicle in uniform circular motion on a banked track. The forces are the weight force,![]() , and the normal reaction force,
, and the normal reaction force, ![]() . The forces are unbalanced and the resulting force is the centripetal force keeping the vehicle in uniform circular motion.
. The forces are unbalanced and the resulting force is the centripetal force keeping the vehicle in uniform circular motion.
The diagrams below break down the weight force and the normal reaction force into horizontal and vertical components:
- Diagram A shows all components
- Diagram B shows the vertical components.
- Diagram C shows the horizontal components
Analysing forces and the equations:
- Using diagram B: the vertical force component =
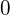 . Therefore:
. Therefore: 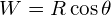
- Using diagram C: the horizontal force component =
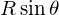 . This is the force that is responsible for the centripetal force, therefore:
. This is the force that is responsible for the centripetal force, therefore: 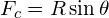
Banked tracks (considering friction):
The diagram below represents the forces acting on a vehicle in uniform circular motion on a banked track, including the friction force. The forces are the weight force,![]() , the normal reaction force,
, the normal reaction force, ![]() and the friction force,
and the friction force, ![]() . The forces are unbalanced and the resulting force is the centripetal force keeping the vehicle in uniform circular motion.
. The forces are unbalanced and the resulting force is the centripetal force keeping the vehicle in uniform circular motion.
The diagrams below break down the weight force, the normal reaction force and the friction force into horizontal and vertical components:
- Diagram A shows all components.
- Diagram B shows the vertical components.
- Diagram C shows the horizontal components
*note: the friction force contributes to the net force in both vertical and horizontal components.
Analysing forces and the equations:
- Using diagram B: the vertical force component =
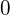 . Therefore:
. Therefore: 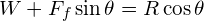
- Using diagram C: the horizontal force component =
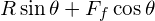 . This is the force that is responsible for the centripetal force, therefore:
. This is the force that is responsible for the centripetal force, therefore: 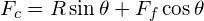
Example 1:
A vehicle with a mass of 1000kg approaches a corner with a radius of curvature of 15m. What is the maximum speed that the car can turn the corner, if:
a) there is a constant friction force of 5000N
b) the coefficient of friction is 0.4
Answers:
a) The friction force provides the centripetal force, therefore: ![]()
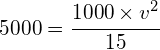
![]()
![]()
![]()
b) ![]()
![]()
![]()
![]()
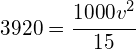
![]()
![]()
Example 2:
A ball with a mass of 0.1kg is swung around in uniform circular motion at an angle of 15° to the vertical as shown below. The length of the string is 0.8m and it makes a circle with a radius of 20.7cm. Calculate:
a) the tension in the string
b) the centripetal force acting on the ball
c) the magnitude of the velocity of the ball
Answers:
a) Using Trig: ![]()
![]()
![]()
![]()
![]()
b) ![]()
Using Trig: ![]()
![]()
![]()
![]()
Therefore: ![]()
c) Using: ![]()
![]()
![]()
![]()
Example 3:
A 800kg car travels around a corner with a radius of 25m in uniform circular motion. The road is banked at 10° as shown below. Calculate:
a) The maximum speed the car can travel around the corner, ignoring friction:
b) The maximum speed the car can travel around the corner when there is a frictional force between the car and the road of 4000N:
Answers:
a) Using: ![]() and first determining the normal reaction force, R:
and first determining the normal reaction force, R:
![]()
![]()
![]()
![]()
Now: ![]()
![]()
![]()
Using: ![]()
![]()
![]()
![]()
b) Using: ![]() and first determining the normal reaction force, R:
and first determining the normal reaction force, R:
![]()
![]()
![]()
![]()
![]()
Now: ![]()
![]()
![]()
![]()
Using: ![]()
![]()
![]()
![]()
