Calculating Magnetic Fields – Learn
The strength of the magnetic field around a current-carrying conductor and a solenoid can be determined quantitatively.
- The equation used to calculate the strength of the field around a current-carrying conductor, gives us the magnitude of the field at some distance, 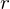r, from the conductor.
- The equation used to calculate the strength of the field in a solenoid, gives us the magnitude of the field at a point inside of the solenoid. The field within the solenoid is uniform.
Current-carrying conductors
The magnitude of the field (\vec { B } ) at some distance, r, from the current-carrying conductor is given by:
\vec { B } =\cfrac { { \mu }_{ 0 }I }{ 2\pi r }
where:
\vec { B } = the strength of the magnetic field (in Tesla)
{ \mu }_{ 0 } = the permeability of free space ({ 1.257\times 10 }^{ -6 }N{ A }^{ -2 } )
I = the current in the wire (in amps)
r = the radius, or distance from the wire (in m)
Solenoids
The magnitude of the field (\vec { B } ) within a solenoid is given by:
\vec { B } =\cfrac { { \mu }_{ 0 }NI }{ L }
where:
\vec { B } = the strength of the magnetic field (in Tesla)
{ \mu }_{ 0 } = the permeability of free space ({ 1.257\times 10 }^{ -6 }N{ A }^{ -2 } )
N = the number of turns in the coil
I = the current in the wire (in amps)
L = the length of the solenoid (in m)
Example 1:
What is the strength of a magnetic field at a distance of 5cm from a wire carrying a current of 10A?
Answer:
\vec { B } =\cfrac { { \mu }_{ 0 }I }{ 2\pi r }
where:
{ \mu }_{ 0 } = ({ 1.257\times 10 }^{ -6 }N{ A }^{ -2 } )
I\:= \:10 \:amps
r\:=\:0.05m
\vec { B } =\cfrac { { { 1.257\times 10 }^{ -6 }\: }\times \: 10 }{ 2\pi \:\times\: 0.05 }
\vec { B } =\:{ 4\times 10 }^{ -5 }
Example 2:
What is the strength of a magnetic field in a 15cm solenoid that has 250 turns carrying a current of 20A?
Answer:
\vec { B } =\cfrac { { \mu }_{ 0 }NI }{ L }
where:
{ \mu }_{ 0 } = ({ 1.257\times 10 }^{ -6 }N{ A }^{ -2 } )
I\:= \:20 \:amps
L\:=\:0.15m
N\:=\:250
\vec { B } =\cfrac { { 1.257\times 10 }^{ -6 }\: \times \: 250\: \times \:20 }{ 0.15 }
\vec { B } = 0.0419\:T