Charges in Magnetic Fields – Learn
When charged particles move through a magnetic field they can experience a force. This force is dependent on several factors:
- The charge on the particle (both negative and positive charges experience a force in an electric field)
- The velocity of the particle
- The magnitude of the magnetic field
- The direction of the moving charge relative to the field. If the particle is moving perpendicular to the field it will experience a maximum force and if it is travelling parallel to the field it will experience no force.
Calculating the Force
The force that a charged particle experiences when it moves through a magnetic field is given by:
![]()
Where:
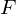 is the force experienced by the charged particle in the magnetic field (in N)
is the force experienced by the charged particle in the magnetic field (in N)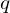 is the electric charge on the particle (in C)
is the electric charge on the particle (in C)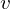 is the velocity as it moves through the magnetic field (in m/s)
is the velocity as it moves through the magnetic field (in m/s)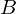 is the strength of the magnetic field (in T)
is the strength of the magnetic field (in T)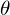 is the angle that the particle is moving in with respect to the field
is the angle that the particle is moving in with respect to the field
.
Determining the Direction
The direction that a force acts on a charged particle as it moves through a magnetic field is given by the right-hand palm rule. To use the right-hand push rule, position your right hand so that:
- The fingers point in the direction of the magnetic field
- The thumb points in the direction of conventional current flow (this means in the direction of the velocity of positive charges or in the opposite direction to the velocity of negative charges)
- The direction of the force on the particles is directly away from the palm of the hand (the way you would ‘push’)
Motion of a Charged Particle in a Magnetic Field
When a charged particle is moving in a plane which is perpendicular to the magnetic field then the resultant force is always perpendicular to the velocity. This results in the particle undergoing circular motion. The mathematics of a charged particle undergoing circular motion in a magnetic field is the same as any other circular motion.
We can derive an equation for the radius of orbit for the charged particle by equating the force experienced by the charged particle in the magnetic field with the centripetal force:
![]()
This equation is rearranged to give:
![]()
Where:
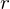 is the radius of the path (in m)
is the radius of the path (in m)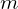 is the mass of the particle (in kg)
is the mass of the particle (in kg)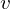 is the velocity of the particle (in m/s)
is the velocity of the particle (in m/s)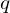 is the charge on the particle (in C)
is the charge on the particle (in C)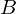 is the strength of the magnetic field (in T)
is the strength of the magnetic field (in T)
Note: ![]() has been omitted because the particle is travelling perpendicular to the field, so
has been omitted because the particle is travelling perpendicular to the field, so ![]() = 1.
= 1.
Example 1:
A positively charged particle with a charge of 5 × 10−18 C enters a magnetic field with a strength of 1.5 × 10−4 T. The particle is travelling at 5000 m/s at an angle of 60° to the magnetic field. Calculate the force experienced by the particle:
Answer:
Using: ![]()
Where:
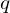 = 5 × 10−18 C
= 5 × 10−18 C 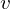 = 5000 m/s
= 5000 m/s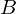 = 1.5 × 10−4 T
= 1.5 × 10−4 T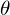 = 60°
= 60°
![]()
![]()
Example 2:
An electron travelling at 2.5 × 106 m/s enters a magnetic field of 0.008 T. What is the radius of its path?
Answer:
Using: ![]()
Where:
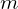 = 9.109 × 10−31 kg
= 9.109 × 10−31 kg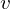 = 2.5 × 106 m/s
= 2.5 × 106 m/s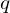 = 1.6 × 10−19 C
= 1.6 × 10−19 C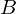 = 0.008 T
= 0.008 T

![]()
![]()
