The Motor Effect - Learn

The Motor Effect – Learn

The motor effect is the resultant force experienced by a current carrying conductor in an external magnetic field.

When the current-carrying conductor passes through or is exposed to an external magnetic field, the magnetic field of the conductor interacts with the external magnetic field and the conductor experiences a force.

The direction of the force on the current-carrying conductor in an external magnetic field can be determined using the right hand palm rule. Using the right hand palm rule:

Thumb points in the direction of the current in the conductor
Fingers point in the direction of the external magnetic field
Open palm indicates the direction of the force acting on the conductor

Factors affecting the magnitude of the force

The magnitude of the force on a straight conductor in a magnetic field depends on the following factors:

The strength of the external magnetic field. The force is proportional to the magnetic field strength, $B$
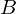
The magnitude of the current in the conductor. The force is proportional to current, $I$
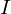
The length of the conductor in the field. The force is proportional to the length, $l$
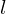
The angle between the conductor and the external magnetic field.

The force is a maximum when the conductor is at right angles to the field, and it is zero when the conductor is parallel to the field.

Calculating the force

To determine the force on the current-carrying conductor in an external magnetic field:

$F=BIl\sin { \theta }$

Where:

$F$ is the force acting on the conductor (in N)

$B$ is the strength of the external magnetic field (in T)

$I$ is the current flowing through the conductor (in A)

$l$ is the length of the conductor (in m)

$\theta$ is the angle between the conductor and the external magnetic field

Example 1:

A conductor with a length of 25cm carries a current of 50 mA. Calculate the magnitude of the force acting on it when it is in a magnetic field with a strength 0.4 T if:

a) the conductor is at right angles to the field

b) the conductor makes an angle of 20° with the field

c) the conductor is parallel with the field.

Answers:

Using: $F=BIl\sin { \theta }$

a) $F=0.4\times 0.05\times 0.25\times \sin { { 90 }^{ \circ } }$

$F=0.005\:N$

b) $F=0.4\times 0.05\times 0.25\times \sin { { 20 }^{ \circ } }$

$F=0.0017\: N$

c) $F=0.4\times 0.05\times 0.25\times \sin { { 0 }^{ \circ } }$

$F=0\: N$